https://www.gregegan.net/SCIENCE/Steffen/Steffen.html
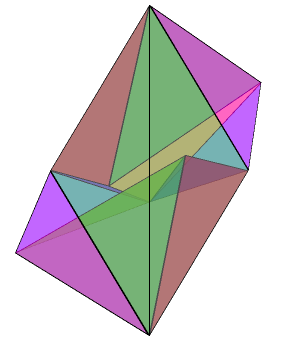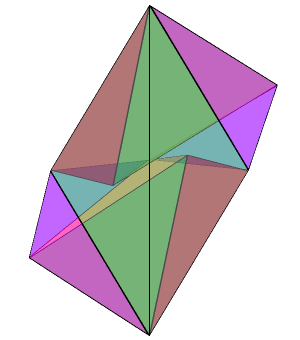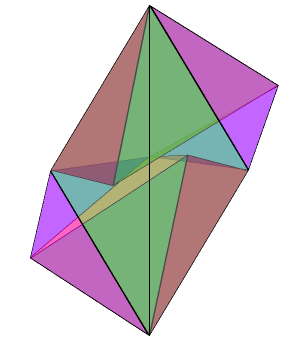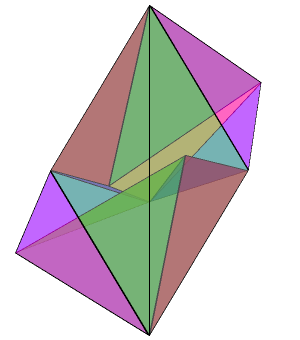
Steffen’s polyhedron is a flexible polyhedron: a polyhedron that can change shape while maintaining the shapes of all its individual faces. It has been proved that no convex polyhedron can be flexible. The first non-self-intersecting flexible polyhedron with the topology of a sphere was found by Robert Connelly in 1977, but Klaus Steffen’s version has fewer faces, with just 14 triangles, and has apparently been proved by Maksimov to be the simplest possible flexible polyhedron with triangular faces.